GATE-XE-2013-E-9-td-1mark
Which of the following processes, shown in the figure below, represents the throttling of an ideal gas?
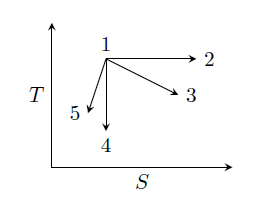
- 1 to 2
- 1 to 3
- 1 to 4
- 1 to 5
During Joule-Thomson expansion of gases,
The relationship \((\partial T/\partial P)_H = 0\) holds good for:
At the inversion point, Joule-Thomson coefficient is:
High pressure steam is expanded adiabatically and reversibly through a well insulated turbine which produces some shaft work. If the enthalpy change and entropy change across the turbine are represented by \(\Delta H\) and \(\Delta S\), respectively, for this process:
Steam undergoes isentropic expansion in a turbine from 5000 kPa and 400\(^\circ\)C (entropy = 6.65 kJ/kg.K) to 150 kPa (entropy of saturated liquid = 1.4336 kJ/kg.K, entropy of saturated vapor = 7.2234 kJ/kg.K). The exit condition of steam is
A saturated liquid at 1500 kPa and 500 K, with an enthalpy of 750 kJ/kg, is throttled to a liquid-vapor mixture at 150 kPa and 300 K. At the exit conditions, the enthalpy of the saturated liquid is 500 kJ/kg and the enthalpy of the saturated vapor is 2500 kJ/kg. The percentage of the original liquid, which vaporizes, is
In a throttling process, the pressure of an ideal gas reduces by 50%. If \(C_P\) and \(C_V\) are the heat capacities at constant pressure and constant volume, respectively (\(\gamma = C_P/C_V\)), the specific volume will change by a factor of
In a steady state steady flow process taking place in a device with a single inlet and single outlet, the work done per unit mass flow rate is given by \(\displaystyle W = -\int _{\text {inlet}}^{\text {outlet}} V dP\), where \(V\) is the specific volume and \(P\) is the pressure. The expression for \(W\) given above
Steam enters an adiabatic turbine operating at steady state with an enthalpy of 3251.0 kJ/kg and leaves as a saturated mixture at 15 kPa with quality (dryness fraction) 0.9. The enthalpies of the saturated liquid and vapor at 15 kPa are \(H_f=225.94\) kJ/kg and \(H_g=2598.3\) kJ/kg respectively. The mass flow rate of steam is 10 kg/s. Kinetic and potential energy changes are negligible. The power output of the turbine in MW is
Which of the following processes, shown in the figure below, represents the throttling of an ideal gas?

A steam turbine operates with a superheated steam flowing at 1 kg/s. This steam is supplied at 41 bar and 500oC, and discharges at 1.01325 bar and 100oC .
Data:
| 41 bar, 500oC | Enthalpy: | 3443.9 kJ/kg |
| Entropy: | 7.0785 kJ/(kg.K) | |
| 41 bar, 251.8oC | Enthalpy of saturated steam: | 2799.9 kJ/kg |
| Entropy of saturated steam: | 6.0583 kJ/(kg.K) | |
| 1.01325 bar, 100oC | Enthalpy of saturated vapor: | 2676 kJ/kg |
| Enthalpy of saturated liquid: | 419.1 kJ/kg | |
| Entropy of saturated vapor: | 7.3554 kJ/(kg.K) | |
| Entropy of saturated liquid: | 1.3069 kJ/(kg.K) |
The maximum power output (in kW) will be ____________
An insulated, evacuated container is connected to a supply line of an ideal gas at pressure \(P_s\). temperature \(T_s\) and specific volume \(V_s\). The container is filled with the gas until the pressure in the container reaches \(P_s\). There is no heat transfer between the supply line to the container, and the kinetic and potential energies are negligible. If \(C_P\) and \(C_V\) are the heat capacities at constant pressure and constant volume, respectively (\(\gamma =C_P/C_V\)), then the final temperature of the gas in the container is
A pump handling a liquid raises its pressure from 1 bar to 30 bar. Take the density of the liquid as 990 kg/m3. The isentropic specific work done by the pump in kJ/kg is
Consider the compression of air from \(10^5\) Pa at 27\(^\circ \)C to \(3 \times 10^6\) Pa in an ideal two-stage compressor with intercooling. Assume that the temperature of the air leaving the intercooler is also 27\(^\circ \)C, and the optimum interstage pressure is used. The compressor is water-jacketed and the polytropic exponent \(\delta \) is 1.3 for both stages. Determine the work of compression (in kJ) per kg of air.
Consider a vessel containing steam at 180oC . The initial steam quality is 0.5 and the initial volume of the vessel is 1 m3. The vessel loses heat a constant rate \(\dot {Q}\) under isobaric conditions so that the quality of steam reduces to 0.1 after 10 hours. The thermodynamic properties of water at 180oC are (subscript \(g\): vapor phase; subscript \(f\): liquid phase):
| specific volume: | \(V_g=0.19405\) m3/kg | \(V_f=0.001127\) m3/kg |
|---|---|---|
| specific internal energy: | \(U_g=2583.7\) kJ/kg, | \(U_f=762.08\) kJ/kg |
| specific enthalpy: | \(H_g=2778.2\) kJ/kg, | \(H_f=763.21\) kJ/kg |
The temperature and pressure of air in a large reservoir are 400 K and 3 bar respectively. A converging-diverging nozzle of exit area 0.005 m2 is fitted to the wall of the reservoir as shown in the figure. The static pressure of air at the exit section for isentropic flow through the nozzle is 50 kPa. The characteristic gas constant and the ratio of specific heats of air are 0.287 kJ/kg.K and 1.4 respectively.

(i) The density of air in kg/m3 at the nozzle exit is
{#1}
(ii) The mass flow rate of air through the nozzle in kg/s is
{#2}
Air enters an adiabatic nozzle at 300 kPa and 500 K, with a velocity of 10 m/s. It leaves the nozzle at 100 kPa with a velocity of 180 m/s. The inlet area is 80 cm2. The specific heat of air \(C_P\) is 1008 J/kg.K.
(i) The exit temperature of air is
{#1}
(ii) The exit area of the nozzle in cm2 is
{#2}
Saturated water vapor enters an adiabatic turbine at 0.8 MPa and leaves at 0.1 MPa. The mass flow rate of water vapor is 25 kg/s. Use the following data table to answer the following questions.
| Pressure | Temperature | Specific enthalpy | Specific entropy | ||
| (MPa) | (\(^\circ\)C) | \(H_L\) (kJ/kg) | \(H_V\) (kJ/kg) | \(S_L\) (kJ/kg.K) | \(S_V\) (kJ/kg.K) |
| 0.8 | 170.43 | 722.11 | 2769.10 | 2.0462 | 6.6628 |
| 0.1 | 99.63 | 417.46 | 2675.50 | 1.3026 | 7.3594 |
(i) The quality of steam at the exit of turbine after an isentropic expansion is
{#1}
(ii) If the steam leaves the turbine as saturated vapor, the power produced by the turbine in kW is
{#2}
An insulated rigid tank of volume 1 m\(^3\), is initially empty. The tank is connected to a supply line of air at 2 MPa and 300 K by a valve. The tank is allowed to fill, and the process ends when the pressure in the tank reaches the supply line pressure. Calculate the final temperature of the air in the tank and the mass of air that entered.
If the tank had initially air 300 K and 1 atm pressure, find the final temperature and mass of air added. For air, \(\gamma=1.4\), and molecular weight = 29.
A pure gas flows at a low rate through a well insulated horizontal pipe at high pressure and is throttled to a slightly lower pressure. The equation of state for the system is given as \(P(V-c) = RT\), where \(c\) is a positive constant. Kinetic energy changes are negligible. Prove whether or not the gas temperature rises or falls due to throttling by using the following equation for Joule-Thomson coefficient, \(\mu _{\text {JT}}\), \[ \mu _{\text {JT}} = \left (\dfrac {\partial T}{\partial P}\right )_H = \frac {T\left (\dfrac {\partial V}{\partial T}\right )_P - V}{C_P} \]
Methane gas is compressed in a steady state flow process from 101 kPa and 27\(^\circ\)C to 500 kPa and 165\(^\circ\)C. Assume methane to be an ideal gas under all conditions [\(R\) = 8.314 J/mol.K; specific heat capacity, \(C_P/R = 1.7 + 0.009 T\text{(K)}\)] and surroundings to be at a constant temperature of 27\(^\circ\)C. If the total entropy change (of the system and surroundings) during the process is 4.5 J/mol.K, find
The specific enthalpy and specific entropy changes of methane.
The net shaft work done and heat exchanged with the surroundings, per mole of methane.
The thermodynamic efficiency of the process.
Last Modified on: 04-May-2024
Chemical Engineering Learning Resources - msubbu
e-mail: learn[AT]msubbu.academy
www.msubbu.in