0600-2-mt-1mark

Sherwood number in mass transfer is analogous to the following dimensionless group in heat transfer

• Graetz number

• Grashoff number

• Nusselt number

• Prandtl number

GATE-CH-1990-5-i-mt-2mark

In the absorption of a solute gas from a mixture containing inerts in a solvent, it has been found that the overall gas transfer coefficient is nearly equal to the individual gas film transfer coefficient. It may therefore be concluded that:

• the process is liquid film controlled

• the gas is sparingly soluble in the solvent

• the transfer rate can be increased substantially by reducing the thickness of the liquid film

• the transfer rate can be increased substantially by reducing the thickness of the gas film

GATE-CH-1994-1-p-mt-1mark

Mass transfer coefficient, \(k\), according to penetration theory varies with mass diffusivity as

• \(D^{0.5}\)

• \(D\)

• \(1/D\)

• \(D^{1.5}\)

GATE-CH-1995-2-p-mt-2mark

For absorbing sparingly soluble gas in a liquid

• gas side mass transfer coefficient should be increased

• liquid side mass transfer coefficient should be increased

• liquid side mass transfer coefficient should be decreased

• mass transfer coefficient must be kept constant

GATE-CH-1997-1-16-mt-1mark

For turbulent mass transfer in pipes, the Sherwood number depends upon the Reynolds number(\(\text {Re}\)) as

• \(\text {Re}^{0.33}\)

• \(\text {Re}^{0.53}\)

• \(\text {Re}^{0.83}\)

• \(\text {Re}\)

GATE-CH-1997-2-13-mt-2mark

According to the film theory of mass transfer, the mass transfer coefficient is proportional to (where \(D\) is the molecular diffusivity)

• \(D\)

• \(D^2\)

• \(D^{0.5}\)

• \(1/D\)

GATE-CH-1998-1-16-mt-1mark

The mass transfer coefficient for a solid sphere of radius ‘\(a\)’, dissolving in a large volume of quiescent liquid, in which \(D\) is the diffusivity of the solute, is

• \(D/a\)

• \(D/(2a)\)

• proportional to \(D^{0.5}\)

• dependent on the Reynold’s number

GATE-CH-1998-1-17-mt-1mark

In an interphase mass transfer process, the lesser the solubility of a given solute in a liquid, the higher are the chances that the transfer process will be

• liquid phase resistance controlled

• gas phase resistance controlled

• impossible

• driven by a nonlinear driving force

GATE-CH-1998-2-14-mt-2mark

If the Prandtl number is greater than the Schmidt number,

• the thermal boundary layer lies inside the concentration boundary layer

• the concentration boundary layer lies inside the thermal boundary layer

• the thermal and concentration boundary layers are of equal thickness

• the hydrodynamic (i.e., momentum) boundary layer is thicker than the other two

GATE-CH-1998-2-19-mt-2mark

In an interphase heat transfer process, the equilibrium state corresponds to equality of temperatures in the two phases, while the condition for equilibrium in an interpahse mass transfer process is

• equality of concentrations

• equality of chemical potentials

• equality of activity coefficients

• equality of mass transfer coefficients

GATE-CH-1999-1-19-mt-1mark

Penetration theory states that the mass transfer coefficient is equal to (where \(D_e\) is diffusivity and \(t\) is time)

• \((D_e t)^{1/2}\)

• \((D_e/t)^{1/2}\)

• \((4D_e/(\pi t))^{1/2}\)

• \((4D_e t)^{1/2}\)

GATE-CH-2001-1-11-mt-1mark

The surface renewal frequency in Danckwerts’ model of mass transfer is given by (\(k_L\): mass transfer coefficient, m/s)

• \(\sqrt {k_L^2D_A}\)

• \(k_L^2D_A\)

• \(k_L^2/D_A\)

• \(k_L/D_A^2\)

GATE-CH-2001-1-12-mt-1mark

For gas absorption the height of a transfer unit, based on the gas phase, is given by (\(G\): superficial molar gas velocity; \(L\): superficial molar liquid velocity; \(F_G\): mass transfer coefficient, mol/m².s; \(a\): interfacial area per unit volume of tower)

• \(\displaystyle \frac {G}{F_Ga}\)

• \(\displaystyle \frac {F_G}{Ga}\)

• \(\displaystyle \frac {Ga}{F_G}\)

• \(\displaystyle \frac {L}{F_GG}\)

GATE-CH-2002-1-24-mt-1mark

The dimensionless group in mass transfer that is equivalent to Prandtl number in heat transfer is

• Nusselt number

• Sherwood number

• Schmidt number

• Stanton number

GATE-CH-2002-1-25-mt-1mark

The Reynolds analogy for momentum, heat and mass transfer is best applicable for

• Gases in turbulent flow

• Gases in laminar flow

• Liquids in turbulent flow

• Liquids and gases in laminar flow

GATE-CH-2003-64-mt-2mark

The Reynolds number of the liquid was increased 100 fold for a laminar film used for gas-liquid contacting. Assuming penetration theory is applicable, the fold-increase in the mass transfer coefficient (\(k_c\)) for the same system is

• 100

• 10

• 5

• 1

GATE-CH-2005-21-mt-1mark

For turbulent flow past a flat plate, when no form drag is present, the friction factor \(f\) and the Chilton-Colburn factor \(j_D\) are related as

• \(f\) and \(j_D\) cannot be related

• \(f\) is equal to \(j_D\)

• \(f\) is greater than \(j_D\)

• \(f\) is less than \(j_D\)

GATE-CH-2005-65-mt-2mark

Match the variation of mass transfer coefficient given by the theory in Group I with the appropriate variation in Group II.

Group I	Group II
(P) Film Theory	(1) \(\propto D_{AB}\)
(Q) Penetration theory	(2) \(\propto D_{AB}^{2/3}\)
(R) Boundary layer theory	(3) \(\propto D_{AB}^{1/2}\)

• P-1, Q-2, R-3

• P-2, Q-1, R-3

• P-1, Q-3, R-2

• P-3, Q-2, R-1

GATE-CH-2006-43-mt-2mark

Experiments conducted with a sparingly dissolving cylinder wall in a flowing liquid yielded the following correlation for the Sherwood number \[ \text {Sh} = 0.023 (\text {Re})^{0.83} \ (\text {Sc})^{1/3} \] Assuming the applicability of the Chilton-Colburn analogy, the corresponding correlation for heat transfer is

• \(\text {St} = 0.023(\text {Gr})^{0.83} \ (\text {Pr})^{1/3}\)

• \(\text {Nu} = 0.023(\text {Re})^{0.83} \ (\text {Pr})^{1/3}\)

• \(j_H = 0.023(\text {Re})^{0.83} \ (\text {Pr})^{2/3}\)

• \(\text {Nu} = 0.069(\text {We})^{0.5} \ (\text {Pr})^{4/3}\)

GATE-CH-2010-3-mt-1mark

The ratio of the thermal boundary layer thickness to the concentration boundary layer thickness is proportional to

• Nu

• Le

• Sh

• Pr

GATE-CH-2011-20-mt-1mark

Simultaneous heat and mass transfer is occurring in a fluid flowing over a flat plate. The flow is laminar. The concentration boundary layer will COINCIDE with the thermal boundary layer, when

• Sc = Nu

• Sh = Nu

• Sh = Pr

• Sc = Pr

GATE-CH-2011-40-mt-2mark

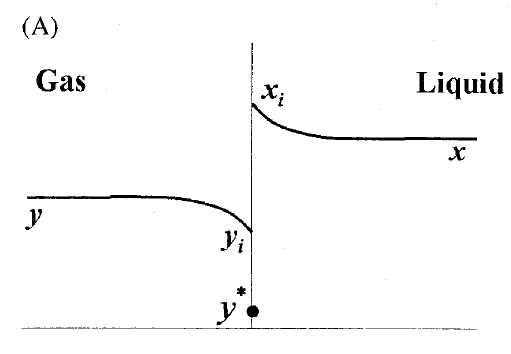
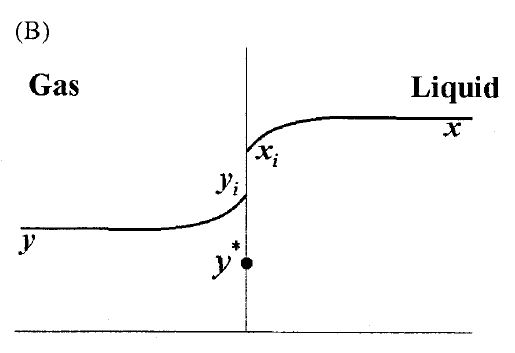
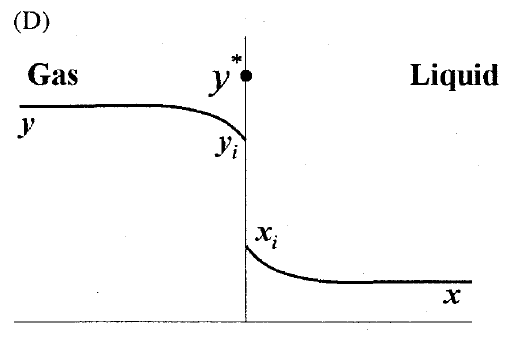
A gas mixture is in contact with a liquid. Component \(P\) in the gas mixture is highly soluble in the liquid. Possible concentration profiles during absorption of \(P\) are shown in choices, where

\(x\) : mole fraction of \(P\) in bulk liquid
\(y\) : mole fraction of \(P\) in bulk gas
\(x_i\) : mole fraction of \(P\) at the interface in liquid
\(y_i\) : mole fraction of \(P\) at the interface in gas
\(y^*\) : equilibrium gas phase mole fraction corresponding to \(x_i\)

The CORRECT profile is

• 
• 
• 
• 

GATE-CH-2012-18-mt-1mark

For which of the following operations, does the absorption operation become gas-film controlled?

• [P.] The solubility of gas in the liquid is very high
• [Q.] The solubility of gas in the liquid is very low
• [R.] The liquid-side mass transfer coefficient is much higher than the gas-side mass transfer coefficient
• [S.] The liquid-side mass transfer coefficient is much lower than the gas-side mass transfer coefficient

• P & Q

• P & R

• P & S

• Q & R

GATE-CH-2014-19-mt-1mark

Assuming the mass transfer coefficients in the gas and the liquid phases are comparable, the absorption of CO₂ from reformer gas (CO₂+H₂) into an aqueous solution of diethanolamine is controlled by

• gas phase resistance

• liquid phase resistance

• both gas and liquid phase resistances

• composition of the reformer gas

GATE-CH-2014-20-mt-1mark

Which ONE of the following statements is CORRECT for the surface renewal theory?

• Mass transfer takes place at steady state

• Mass transfer takes place at unsteady state

• Contact time is same for all the liquid elements

• Mass transfer depends only on the film resistance

GATE-CH-2016-12-mt-1mark

Match the dimensionless numbers in Group-1 with the ratios in Group-2.

Group-1	Group-2
P. Biot number	I. \(\dfrac {\text {buoyancy force}}{\text {viscous force}}\)
Q. Schmidt number	II. \(\dfrac {\text {internal thermal resistance of a solid}}{\text {boundary layer thermal resistance}}\)
R. Grashof number	III. \(\dfrac {\text {momentum diffusivity}} {\text {mass diffusivity}}\)

• P-II, Q-I, R-III

• P-I, Q-III, R-II

• P-III, Q-I, R-II

• P-II, Q-III, R-I

GATE-CH-2017-16-mt-1mark

Consider steady state mass transfer of a solute \(A\) from a gas phase to a liquid phase. The gas phase bulk and interface mole fractions are \(y_{A,G}\) and \(y_{A,i}\), respectively. The liquid phase bulk and interface mole fractions are \(x_{A,L}\) and \(x_{A,i}\), respectively. The ratio \(\dfrac {(x_{A,i}-x_{A,L})}{(y_{A,G}-y_{A,i})}\) is very close to zero. This implies that mass transfer resistance is

• negligible in the gas phase only

• negligible in the liquid phase only

• negligible in both the phases

• considerable in both the phases

GATE-CH-2017-43-mt-2mark

The Sherwood number (Sh\(_{L}\)) correlation for laminar flow over a flat plate of length \(L\) is given by \[ \text {Sh}_{L} = 0.664\; \text {Re}_L^{0.5}\; \text {Sc}^{1/3} \] where Re\(_L\) and Sc represent Reynolds number and Schmidt number, respectively. This correlation, expressed in the form of Chilton-Colburn \(j_D\) factor, is

• \(j_D=0.664\)

• \(j_D=0.664\; \text {Re}_L^{-0.5}\)

• \(j_D=0.664\; \text {Re}_L\)

• \(j_D=0.664\; \text {Re}_L^{0.5}\; \text {Sc}^{2/3}\)

GATE-CH-1994-3-k-mt-1mark

Forced convection is relatively more effective in increasing the rate of mass transfer if Schmidt number is larger. (True/False)

• True
• False

GATE-CH-1994-20-mt-5mark

A stream of air at 100 kPa pressure and 300 K is flowing on the top surface of a thin flat sheet of solid naphthalene of length 0.2 m with a velocity of 20 m/s.
The other data are:
Mass diffusivity of naphthalene vapor in air = \(6 \times 10^{-6}\) m\(^2\)/s
Kinematic viscosity of air = \(1.5 \times 10^{-5}\) m\(^2\)/s
Concentration of naphthalene at the air-solid naphthalene interface = \(1 \times 10^{-5}\) kmol/m\(^3\)

Note: For heat transfer over a flat plate, convective heat transfer coefficient for laminar flow can be calculated by the equation: \[ \text {Nu} = 0.664 \text {Re}_L^{1/2} \text {Pr}^{1/3} \] You may use analogy between mass and heat transfer.
Calculate:
(a) the average mass transfer coefficient( in m/s) over the flat plate
{#1}

(b) the rate of loss of naphthalene from the surface per unit width (in mol.m\(^{-1}\).h\(^{-1}\))
{#2}

GATE-CH-2004-19-20-mt-2mark

Pure aniline is evaporating through a stagnant air film of 1 mm thickness at 300 K and a total pressure of 100 kPa. The vapor pressure of aniline at 300 K is 0.1 kPa. The total molar concentration under these conditions is 40.1 mol/m³. The diffusivity of aniline in air is \(0.74 \times 10^{-5}\) m²/s.

(i) The numerical value of the mass transfer coefficient is \(7.4\times 10^{-3}\). Its units are

{#1}

(ii) The rate of evaporation of aniline is \(2.97 \times 10^{-4}\). Its units are

{#2}

GATE-CH-2000-2-14-mt-2mark

The individual mass transfer coefficients (mol/m².s) for absorption of a solute from a gas mixture into a liquid solvent are \(k_x\) =4.5 and \(k_y\) = 1.5. The slope of the equilibrium line is 3. Which of the following resistance(s) is (are) controlling?

• liquid-side

• gas-side

• interfacial

• both liquid and gas side

GATE-CH-2001-2-12-mt-2mark

The interfacial area per unit volume of dispersion, in a gas-liquid contactor, for fractional hold-up of gas = 0.1 and gas bubble diameter = 0.5 mm is given by (in m²/m³)

• 500

• 1200

• 900

• 800

GATE-CH-2005-64-mt-2mark

Two solid discs of benzoic acid (molecular weight = 122) of equal dimensions are spinning separately in large volumes of water and air at 300 K. The mass transfer coefficients for benzoic acid in water and air are \(0.9 \times 10^{-5}\) and \(0.47 \times 10^{-2}\) m/s respectively. The solubility of benzoic acid in water is 3 kg/m³ and the equilibrium vapor pressure of benzoic acid in air is 0.04 kPa. Then the disc

• dissolves faster in air than in water

• dissolves faster in water than in air

• dissolves at the same rate in both air and water

• does not dissolve either in water or in air

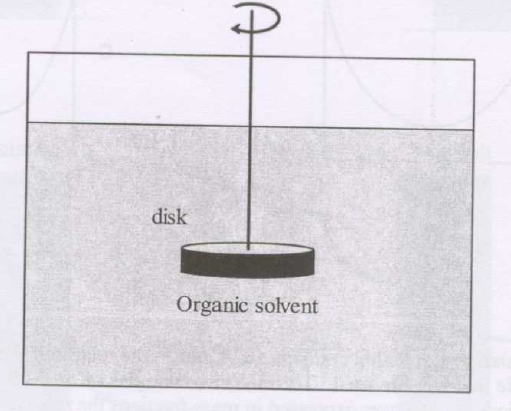
GATE-CH-2008-52-mt-2mark

A sparingly soluble solute in the form of a circular disk is dissolved in an organic solvent as shown in the figure. The area available for mass transfer from the disk is \(A\) and the volume of the initially pure organic solvent is \(V\). The disk is rotated along the horizontal plane at a fixed rpm to produce a uniform concentration of the dissolving solute in the liquid. The convective mass transfer coefficient under these conditions is \(k_c\). The equilibrium concentration of the solute in the solvent is \(C^*\). The time required for the concentration to reach 1% of the saturation value is given by

• \({\displaystyle \exp \left (-\frac {k_cA}{V}t\right )=0.99}\)

• \({\displaystyle \exp \left (-\frac {k_cA}{V}t\right )=0.01}\)

• \({\displaystyle \frac {V}{Ak_c} \exp (-0.99) = t}\)

• \({\displaystyle \frac {V}{Ak_c} \exp (0.01) = t}\)

GATE-CH-2014-31-mt-2mark

A spherical ball of benzoic acid (diameter = 1.5 cm) is submerged in a pool of still water. The solubility and diffusivity of benzoic acid in water are 0.03 kmol/m³ and \(1.25 \times 10^{-9}\) m²/s respectively. Sherwood number is given as \(\text {Sh} = 2.0 + 0.6 \text {Re}^{0.5}\text {Sc}^{0.33}\). The initial rate of dissolution (in kmol/s) of benzoic acid approximately is

• \(3.54\times 10^{-11}\)

• \(3.54\times 10^{-12}\)

• \(3.54\times 10^{-13}\)

• \(3.54\times 10^{-14}\)

GATE-CH-1991-6-i-mt-2mark

The diffusion rate of ammonia from an aq.solution to the gas phase is \(10^{-3}\) kmol/m\(^2\).s. The interface equilibrium pressure of \(\ce {NH3}\) is 660 N/m\(^2\) and the concentration of \(\ce {NH3}\) in the gas phase is 5%. If the total pressure is 101 N/m\(^2\), temperature is 295 K and diffusivity of \(\ce {NH3}\) is 0.24 cm\(^2\)/s, the gas film thickness is ____________(\(\mu \)m).

• Answer

GATE-CH-2001-13-mt-5mark

A sugary substance \(A\) is added to a pot of milk (initially containing no \(A\)) and stirred vigorously by a spoon so that the concentration of \(A\), \(C_A\), is uniform everywhere. The mass transfer coefficient for the transfer of \(A\) into the liquid is \(k_{sl} = 1\times10^{-4}\) m/s. Solid \(A\) is added in great excess compared to the saturation capacity of milk to dissolve \(A\). Assume that the solid-liquid interfacial area stays constant throughout the dissolution process and is given by \(a=1000\) cm\(^2\). Derive the expression for \(C_A\) versus time, \(t\). The time (in s) taken for \(C_A/C_A^*=0.95\) ___________

  \(C_A^* = 5\times10^{-2}\) kmol/m\(^3\); \(V_L\) = 1000 cm\(^3\).

• Answer

GATE-CH-2002-12-mt-5mark

The mass flux from a 5 cm diameter naphthalene ball, placed in stagnant air at 40\(^\circ\)C and atmospheric pressure, is \(1.47 \times 10^{-3}\) mol/m\(^2\).s. Assume the vapor pressure of naphthalene to be 0.15 atm at 40\(^\circ\)C and negligible bulk concentration of naphthalene in air. If air starts blowing across the surface of naphthalene ball at 3 m/s, by what factor will the mass transfer rate increase, all other conditions remaining the same? _______________

For spheres: \[\text{Sh} = 2.0 + 0.6 (\text{Re})^{0.5} (\text{Sc})^{0.33}\] where \(\text{Sh}\) is the Sherwood number and \(\text{Sc}\) is the Schmidt number. The viscosity and density of air are \(1.8\times10^{-5}\) kg/m.s and 1.123 kg/m\(^3\), respectively and the gas constant is 82.06 cm\(^3\).atm/mol.K

• Answer

GATE-CH-2013-39-mt-2mark

A study was conducted in which water was pumped through cylindrical pipes made of a sparingly soluble solid. For a given pipe and certain flow conditions, the mass transfer coefficient \(k_c\) has been calculated as 1 mm/s using the correlation \(\text {Sh} = 0.025 \text {Re}^{0.6} \text {Sc}^{0.33}\). If the velocity of the fluid and the diameter of the pipe are both doubled, what is the new value of \(k_c\) in mm/s, up to 2 digits after the decimal point? ____________

• Answer

GATE-CH-1990-15-iii-mt-6mark

Consider a system in which component \(A\) is being transferred from a gas phase to a liquid phase. The equilibrium relation is given by \(y_A = 0.75 x_A\) where \(y_A\) and \(x_A\) are mole fractions of \(A\) in gas and liquid phase respectively. At one point in the equipment, the gas contains 10 mole % \(A\) and liquid 2 mole % \(A\). Gas film mass transfer coefficient \(k_y\) at this point is 10 kmol/(h.m\(^2\).\(\Delta y_A\)) and 60% of the resistance is in the gas film. Calculate:
(a) the overall mass transfer coefficient in kmol/(h.m\(^2\).\(\Delta y_A\)).
{#1}

(b) mass flux of \(A\) in kmol/(h.m\(^2\)).
{#2}

(c) the interfacial gas concentration of \(A\) in mole fraction.
{#3}

GATE-CH-1988-5-a-ii-mt-1mark

A pure gas is absorbed in a solvent in which the gas is highly soluble. The controlling resistance is in the ––––- film.

• Answer

GATE-CH-1989-5-i-b-mt-1mark

Schmidt number is the ratio of ––––- diffusivity to ––––- diffusivity.

• Answer

GATE-CH-1989-5-i-c-mt-1mark

Reynolds analogy between momentum and mass transfer is applicable when ––––- number is equal to ––––- .

• Answer

GATE-CH-1992-6-c-mt-2mark

––––- number in mass transfer corresponds to Nusselt number in heat transfer and ––––- number to Prandtl number.

• Answer

GATE-CH-1993-12-c-mt-2mark

Associate the following dimensionless groups, with heat transfer, mass transfer and momentum transfer.

• Sherwood number
• Prandtl number
• Nusselt number
• Schmidt number

• Answer

GATE-CH-1994-2-o-mt-1mark

The Reynolds analogy for mass transfer is given by ––––- and is applicable when Schmidt number is ––––-

• Answer