GATE-CH-1995-1-q-ctrl-1mark

Bode diagrams are generated from output response of the system subjected to which of the following input

• impulse

• step

• ramp

• sinusoidal

GATE-CH-1995-1-t-ctrl-1mark

According to Bode stability criterion, a system is unstable if the open loop frequency response exhibits an amplitude ratio exceeding unity at frequency for which phase lag is

• \(0^\circ \)

• \(45^\circ \)

• \(90^\circ \)

• \(180^\circ \)

GATE-CH-1998-1-24-ctrl-1mark

A first order system with a time constant of 1 min is subjected to frequency response analysis. At an input frequency of 1 radian/min, the phase shift is

• \(45^{\circ }\)

• \(-90^{\circ }\)

• \(-180^{\circ }\)

• \(-45^{\circ }\)

GATE-CH-1999-1-27-ctrl-1mark

A sinusoidal variation in the input passing through a linear first-order system

• becomes more oscillatory (frequency increases)

• becomes less oscillatory (frequency decreases)

• gets amplified (magnitude increases)

• gets attenuated (magnitude decrease)

GATE-CH-2000-1-27-ctrl-1mark

Select the correct statement from the following:

• The frequency response of a pure capacity process is unbounded
• The phase lag of a pure time delay system decreases with increasing frequency
• The amplitude ratio of a pure capacity process is inversely proportional to the frequency
• The amplitude ratio of a pure time delay system increases with frequency

GATE-CH-2000-1-28-ctrl-1mark

For a feedback control system to be stable, the

• roots of the characteristics equation should be real
• poles of the closed loop transfer function should lie in the left half of the complex plane
• Bode plots of the corresponding open loop transfer function should monotonically decrease
• poles of the closed loop transfer function should lie in the right half of the complex plane

GATE-CH-2002-1-15-ctrl-1mark

A first order system with unity gain and time constant \(\tau\) is subjected to a sinusoidal input of frequency \(\omega=1/\tau\). The amplitude ratio for this system is

• 1

• 0.5

• \(1/\sqrt{2}\)
• 0.25

GATE-CH-2002-2-16-ctrl-2mark

The frequency response of a first order system, has a phase shift with lower and upper bounds given by

• \([-\infty,\pi/2]\)

• \([-\pi/2,\pi/2]\)

• \([-\pi/2,0]\)

• \([0,\pi/2]\)

GATE-CH-2010-24-ctrl-1mark

The transfer function, \(G(s)\), whose asymptotic Bode diagram is shown below, is

• \(10s+1\)

• \(s-10\)

• \(s+10\)

• \(10s-1\)

GATE-CH-2012-25-ctrl-1mark

The Bode stability criterion is applicable when

• Gain and phase curves decreases continuously with frequency
• Gain curve increases and phase curve decreases with frequency
• Gain curve and phase curve both increases with frequency
• Gain curve decreases and phase curve increases with frequency

GATE-CH-1992-19-b-ctrl-6mark

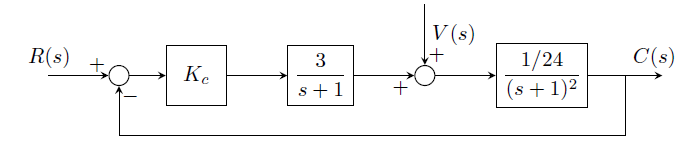
For the loop above, determine: 

(i) The maximum gain for stable operation.
{#1}

(ii) The corresponding frequency of oscillation (rad/min).
{#2}

GATE-CH-1997-26-ctrl-2mark

The open loop transfer function for a process is \(\dfrac {1}{4(3s+1)^4}\), where the time constant is in minutes.
Determine: 

(i) the crossover frequency (rad/min)
{#1}

(ii) the ultimate gain.
{#2}

GATE-CH-2000-14-ctrl-5mark

A feedback control loop with a proportional controller has an open loop transfer function \(G_L(s) = \dfrac {K_c}{s(5s+1)^2}\) where time is in minutes.

(i) The crossover frequency in radians/min = _________

{#1}

(ii)The ultimate controller gain = _________

{#2}

GATE-CH-2006-84-85-ctrl-4mark

For the system shown below, \(G_1(s) = \dfrac {1}{\tau _1s+1}\), \(G_2(s)=\dfrac {1}{\tau _2s+1}\) and \(\tau _2=2\tau _1\).

 
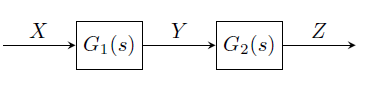
When the system is excited by the sinusoidal input \(X(t) = \sin \omega t\), the intermediate response \(Y\) is given by \( Y = A\sin (\omega t+\phi )\). 

(i) If the response \(Y\) lags behind the input \(X\) by 45\(^\circ \) and \(\tau _1=1\), then the input frequency \(\omega \) is
{#1}

(ii) For the same input, the amplitude of the output \(Z\) will be
{#2}

GATE-CH-1998-2-21-ctrl-2mark

The frequency response of a dynamic element shows a constant magnitude ratio at all frequencies. The element exhibits a negative phase shift at all frequencies. The absolute value of the phase shift increases linearly with frequency. The element has the transfer function

• \(e^{-\tau s}\)

• \(\dfrac {\tau _1s+1}{\tau _2s+1}\)

• \(\dfrac {\tau _1s}{\tau _2s+1}\)

• \(\dfrac {\tau _1s+1}{\tau _2s}\)

GATE-CH-2000-2-23-ctrl-2mark

The time constant of a unity gain, first order plus time delay process is 5 min. If the phase lag at a frequency of 0.2 rad/min is 60^o, then the dead time (in minutes) is

• \(5\pi/12\)
• \(\pi/6\)
• \(\pi/12\)
• \(\pi/3\)

GATE-CH-2001-2-18-ctrl-2mark

An ideal PID controller has the transfer function \(\left[1+\dfrac{1}{0.5s} + 0.2s\right]\). The frequency at which the magnitude ratio of the controller is 1, is

• 0.5/0.2

• 0.2/0.5

• 0.2\(\times\)0.5

• \(1/\sqrt{0.2\times0.5}\)

GATE-CH-2003-77-ctrl-2mark

Find the ultimate gain and frequency for a proportional controller in the case of a process having the following transfer function

\[ G_p(s) = \frac{1}{(4s+1)(2s+1)(s+1)} \]

• \(\displaystyle \omega = \frac{1}{\sqrt{14}}; \ K_c = \frac{45}{7\sqrt{14}}\)

• \(\displaystyle \omega = \sqrt{\frac{7}{6}}; \ K_c = \frac{46}{3}\)

• \(\omega = 1; \ K_c = 13\)

• \(\displaystyle \omega = \sqrt{\frac{7}{8}}; \ K_c = \frac{45}{4}\)

GATE-CH-2004-80-ctrl-2mark

Consider a system with open-loop transfer function

\[ G(s) = \frac{1}{(s+1)(2s+1)(5s+1)} \]

Match the range of \(\omega\) (frequency) in Group I with the slope of the asymptote of the \(\log \text{AR}\) (amplitude ratio) versus \(\log \omega\) plot in Group II.

Group I	Group II
(P) \(0 < \omega < 0.2\)	(1) \(\quad -5\)
(Q) \(\omega > 1\)	(2) \(\quad -3\)
	(3) \(\quad -2\)
	(4) \(\quad -1\)
	(5) \(\quad \)zero

• P-5, Q-2

• P-4, Q-2

• P-5, Q-3

• P-4, Q-1

GATE-CH-2005-50-ctrl-2mak

The value of ultimate period of oscillation \(P_u\) is 3 minutes, and that of the ultimate controller gain \(K_{cu}\) is 2. Select the correct set of tuning parameters (controller gain \(K_c\), derivative time constant \(\tau_D\) in minutes, and the integral time constant \(\tau_I\) in minutes) for a PID controller using Ziegler-Nichols controller settings.

• \(K_c=1.1; \quad \tau_I=2.1; \quad \tau_D=1.31\)

• \(K_c=1.5; \quad \tau_I=1.8; \quad \tau_D=0.51\)

• \(K_c=1.5; \quad \tau_I=1.8; \quad \tau_D=0.51\)

• \(K_c=1.2; \quad \tau_I=1.5; \quad \tau_D=0.38\)

GATE-CH-2006-58-ctrl-2mark

A process is perturbed by a sinusoidal input, \(u_t = A \sin \omega t\). The resulting process output is \(Y(s) = \dfrac{K A\omega}{(\tau s+1)(s^2+\omega^2)}\). If \(y(0) = 0\), the differential equation representing the process is

• \(\dfrac{dy(t)}{dt} + \tau y(t) = K u(t)\)

• \(\tau \dfrac{dy(t)}{dt} + y(t) = K Au(t)\)

• \(\tau \dfrac{dy(t)}{dt} + y(t) = K u(t)\)

• \({\displaystyle \tau \left[\dfrac{dy(t)}{dt} + y(t)\right] = K Au(t)}\)

GATE-EE-2013-A-15-ctrl-1mark

The Bode plot of a transfer function \(G(s)\) is shown in the figure below.

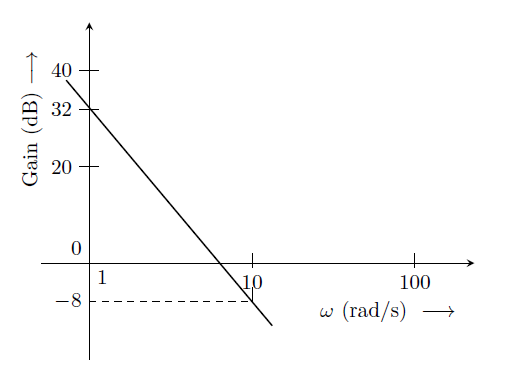
The gain \((20\log |G(s)|)\) is 32 dB and -8 dB at 1 rad/s and 10 rad/s respectively. The phase is negative for all \(\omega\). Then \(G(s)\) is

• \(\dfrac{39.8}{s}\)

• \(\dfrac{39.8}{s^2}\)

• \(\dfrac{32}{s}\)

• \(\dfrac{32}{s^2}\)

GATE-IN-2014-23-ctrl-1mark

A plant has an open-loop transfer function, \[ G_p(s) = \frac{20}{(s+0.1)(s+2)(s+100)} \] The approximate model obtained by retaining only one of the above poles, which is closest to the frequency response of the original transfer function at low frequency is

• \(\dfrac{0.1}{s+0.1}\)
• \(\dfrac{2}{s+2}\)
• \(\dfrac{100}{s+100}\)
• \(\dfrac{20}{s+0.1}\)

GATE-CH-2017-51-ctrl-2mark

The open loop transfer function of a process with a proportional controller (gain \(K_c\)) is \[ G_{OL} = K_c\frac{e^{-2s}}{s} \] Based on Bode criterion for closed-loop stability, the ultimate gain of the controller, rounded to 2 decimal places, is ______

• Answer

GATE-CH-2018-44-ctrl-2mark

Consider the following transfer function: \[ G(s) = \frac{3}{(5s+1)^2} \] where, the natural period of oscillation is in min. The amplitude ratio at a frequency of 0.5 rad/min is ________ (rounded off to second decimal place).

• Answer

GATE-CH-2019-49-ctrl-2mark

For the closed loop system shown in figure, the phase margin (in degrees) is ___________

• Answer

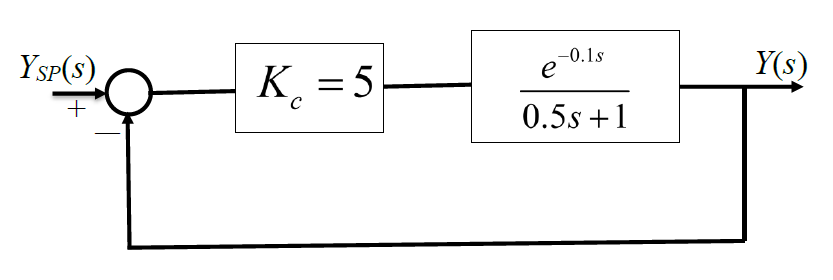
GATE-IN-2014-46-ctrl-2mark

The transfer function of a system is given by \(\displaystyle G(s) = \frac{e^{-s/500}}{s+500}\). The input to the system is \(x(t)=\sin 100\pi t\). In periodic steady state the output of the system is found to be \(y(t)=A\sin(100\pi t - \phi)\). The phase angle (\(\phi\)) in degree is _______

• Answer

GATE-CH-1999-2-18-ctrl-2mark

Each item given in the left-hand column is closely associated with a specific characteristic listed in the right-hand column. Match each of the items with the corresponding characteristic.

• I. Transportation lag

Answer 1D. Unstable responseC. HyperbolicF. Phase angle is $-90^\circ $E. Increase in phase marginB. Phase lag is proportional to frequencyA. Increase in gain margin

• II. Control valve

Answer 2D. Unstable responseC. HyperbolicF. Phase angle is $-90^\circ $E. Increase in phase marginB. Phase lag is proportional to frequencyA. Increase in gain margin